According to SpinCalc, for a centripetal acceleration of 1G the angular velocity (spin rate) of a wheel of radius 50 metres would need to be 4.23 rotations per minute.

If you are sufficiently interested to do some research, William, try asking the web.
Ask: how fast would a wheel have to spin to get earth gravity.

There are several interesting links found.

One is Wikpedia,
https://en.wikipedia.org/wiki/Rotating_ … ce_station

and another is :
https://space.stackexchange.com/questio … -gravity-f

EDIT. Both links are worth reading if you're really interested in the topic. 

There is actually a spin calculator reference in the latter.
http://www.artificial-gravity.com/sw/SpinCalc/

EDIT: (Alfred  found  spin calc and the answer while I was writing this post, and having lunch.)

To answer the question, the formula appears to be : a = ω²r,
and using the spin calculator, to achieve 1 g at a diameter of 100 m, the wheel needs to spin at 4.23 rotations / minute.
(Agrees with my finding)

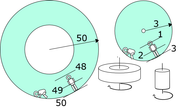
Bear in mind that if the 100m  (radius 50m) is the floor, the outer rim of the " toroidal cabin" of the wheel, and if the astronaut has a height of say 2m, his head will be in a region of gravity at 0.96 g, in other words, lower by 4%.

If the spaceship  was in the form of a spinning cylinder rather than a wheel, and if the floor was the cylindrical skin, say 3m from the long axis, to get the mid height, say 1m of the astronaut at 1g, his feet would be at a increased gravity, and his head much decreased. The three values would be :
at his  midriff radius of 2m,  1g, the spin rate would be 21.15 revs/minute ,
then, at his feet,  radius 3m, gravity would be 1.5 g,
and at  his head, 3.8m, 0.5g

If he had to bend down to pick up something from the floor, and had to get his head near the floor, his head would experience a gravity change of 0.5 to 1.5, or 1 g. Very disorientating. This is why a wheel is the best shape to minimise this effect. harder to launch, it would have to be assembled in space. One of the refs, Wiki?, discusses this problem.

Please check these figures, I took them from the spin calculator in ref 2

Remember that for a constant spin, the gravity value is directly proportional to the radius.

When starting the calculations. I picked on 1.8 m, but changed it to 2 to keep the calculations very simple. I agree, 2 is a bit on the tall side, but if anyone is sufficiently interested to do the calculation, there is enough information to do it. As the centripetal force is directly proportional to the radius, you can see that calculations for1.8 fall short by 1.8/2 = 0.9.or 10 %.
Actually, re the direction of the gravity field, the 1.8 value will be greater by 10%. This does reduce the differences.
I'm not going to revise the text. If William wants the actual values, he can work them out for himself - I presume the question is just curiosity on his part, to start off the thread.

I did say,

Let's say he had to screw a cable in near floor level, under an overhanging equipment drawer. It is only there to make a point about disorientating gravity changes.

Mea culpa. corrected and added material in the edit. By your leave...., or don't leave!